Solution Solution provided by AtoZmathcom Substitution Method Solve Linear Equation in Two Variables Solve linear equation in two variables 1 12x 5y = 7 and 2x 3y 5 = 0 2 x y = 2 and 2x 3y = 4 3 7y 2x 11 = 0 and 3x y 5 = 0SUBSTITUTION METHOD EXAMPLES The following steps will be useful to solve the systems of linear equations using substitution Step 1 In the given two equations, solve one of the equations either for x or y Step 2 Substitute the result of step 1 into other equation and solve for the second variable Step 3Solve for x x in the first equation Tap for more steps Move all terms not containing x x to the right side of the equation Tap for more steps Add 1 1 to both sides of the equation Add 3 3 and 1 1 Divide each term by 2 2 and simplify Tap for more steps Divide each term in 2 x = 4 2 x

Solve The Following Pair Of Linear Equation By Substitution Method I X Y 2 3x 2y 16 Brainly In
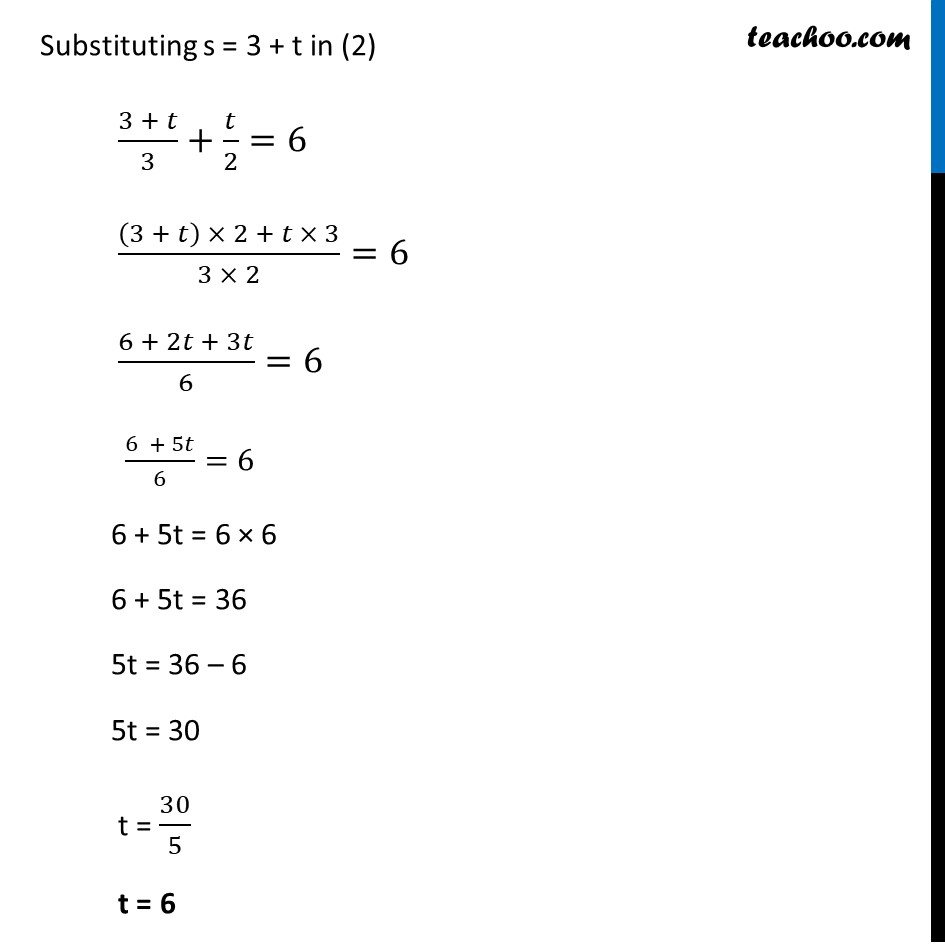
X-y=3 x/3+y/2=6 by substitution method
X-y=3 x/3+y/2=6 by substitution method-Solve for x and y, using substitution method ` 2x y = 7, 4x 3y 1 =0 `You can put this solution on YOUR website!



Ex 3 3 1 Solve By Substitution Method I X Y 14 Ex 3 3
Substitution method can be applied in four steps Step 1 Solve one of the equations for either x = or y = Step 2 Substitute the solution from step 1 into the other equation Step 3 Solve this new equation Step 4 Solve for the second variableAubrey is using the substitution method to solve the following system of equations y − x = 21 2y = 2x 16 She arrives at an answer of 8 = 21 She thinks that this answer means that the lines are parallel and that the system has no solutionHere are the Steps using Substition method 1 In either equation, solve for one variable in terms of the other We are going to solve for y in terms of x xy=12 y = x12 2 Substitute x12 for y in the other equation x3/2y=3/2 Solve x, y = x12 x = 33 3 Substitute the result from step 2 in either equation Solve for the other variable y
Solve by Substitution Calculator Step 1 Enter the system of equations you want to solve for by substitution The solve by substitution calculator allows to find the solution to a system of two or three equations in both a point form and an equation form of the answer Step 2 Click the blue arrow to submitSolve by Substitution y=x6 y=2x3 y = x 6 y = x 6 y = −2x − 3 y = 2 x 3 Eliminate the equal sides of each equation and combine x6 = −2x−3 x 6 = 2 x 3 Solve x6 = −2x−3 x 6 = 2 x 3 for x x Tap for more steps Move all terms containing x x to the left side of the equation One way is to use the method of elimination Step 1 Enter the equations 1 y = x2 3x 2 y = 6 −2x Step 2 Solve for one of the variables in terms of the other 2 y = 6 −2x Since this is already done for us, we can go on to the next step
Let's first solve for 'y' in the 1st equation, then substitute that into 2nd equation 2x y = 3 ==> y = 3 2x 4x y 3 is not an equation, so let's assume you meant 4x y = 3 4x y = 3 ==> 4x (3 2x) = 3 ==> 2x = 6 ==> x = 3 Place x = 3 back into either equation to find 'y', use the 1st equation, for example 2x y = 3 ==> 2 (3) y = 3 ==> y = 9(a) 2x 3y = 12(i) and x y = 1(ii) (ii)×3 ==> 3x 3y = 3(iii) Now we can eliminate y by adding (i) & (iii) (i) (iii) ==> 5x = 15 so x=3 Transcript Ex 33, 1 Solve the following pair of linear equations by the substitution method (i) x y = 14 x – y = 4 x y = 14 x – y = 4 From equation (1) x y = 14 x = 14 – y Substituting value of x in equation (2) x – y = 4 (14 – y) – y = 4 14 – y – y = 4 14 – 2y = 4 –2y = 4 – 14 –2y = –10 y = (−10)/(−2) y = 5 Putting y = 5 in (2) x – y = 4 x = y 4 x



The Substitution Method
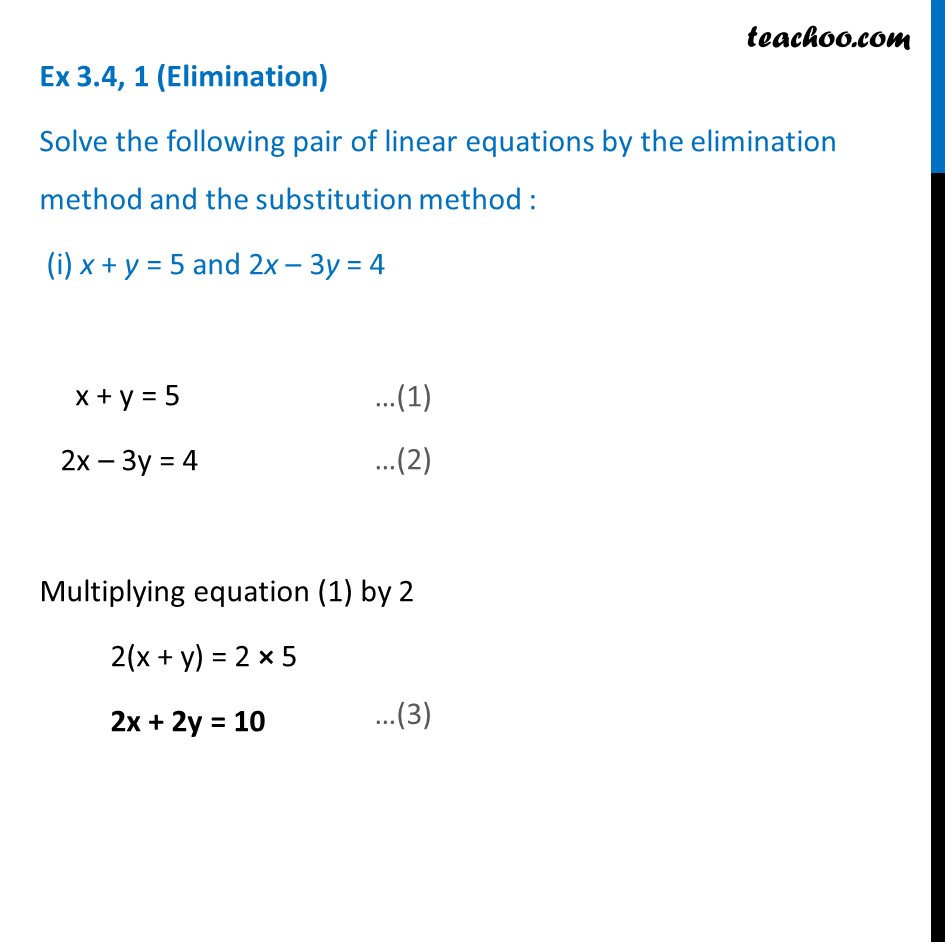



Ex 3 4 1 Solve By Elimination And Substitution I X Y 5 2x 3y
Solve the following pair of linear equations by the substitution method (3x)/2 (5y)/3 = 2, x/yy/2 = 13/6 How do you solve #12y3x=1# and #x4y=1# using the substitution method?By substitution method x y =5 (1) subtract y both side we get x = 5 y ,(4) plug the value of x in equation second we get 2(5 – y ) – 3y = 45y = 6 y = 6/5 = 6/5 Plug the value of y in equation 4 we get x = 5 – 6/5 x = 19/5
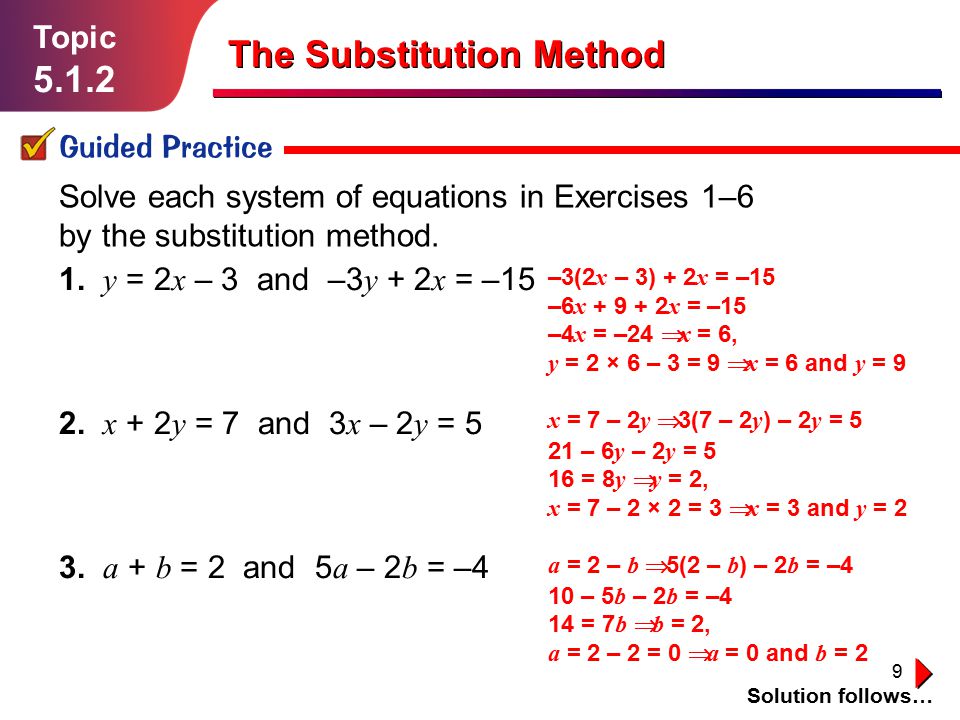



1 Topic The Substitution Method 2 Topic The Substitution Method California Standard 9 0 Students Solve A System Of Two Linear Equations Ppt Download




1 Solve The Following Pair Of Linear Equations By The Substitution Method 1 X Y 14 Ii S T 3 S 6 X Y 4 Ii 3x Y 3 9x 3y 9 3 2 Iv 0 2x 0 3y 1 3 0 4x
3x/2 5y/3 = 2 and x/3y/2=13/6 Solve using substitution methodQuestion 2 Substitution Method Xy=16 And Y=3x 3 Solve The System By The Substitution Method Y= 2x 7 And 2x 3y = 19 4 Xy= 1 And Xy= 5 Solve The System By The Addition Method 5 Solve The System By The Addition Method 4x13y= 8 And 2x13y=4 6 Solve The System By Graphing 3x2y=12 And X 2y= 4 Explanation 2x 3y = 6 x y = 3 Let's solve for x in the second equation x = 3 −y Now let's plug (3 − y) in for x in the first equation 2(3 −y) 3y = 6 6 − 2y 3y = 6 y = 0




Solve The Following System Of Equations 27 X Y 15 X Y 2 And 30 X Y 1 X Y 3 Mathematics Topperlearning Com X68shsoo



Solved Find The Common Solution Of Each Using The Elimination Substitution And Graphical Method 2x Y 2 X 3y 36 2 3x Y 6 X Y 6 3 Course Hero
Use the Substitution method to solve the system of equations y 2x = 5 3y x = 5 Solve one of the equations for x or y Let's solve the first one for y y 2x = 5 y = 2x 5 Now let's substitute 2x 5 for y in the second equation to solve for x 3(2x 5) x = 5 6x 15 x = 5 5x 15 = 5 5x = x = 4 Substitute 4 for x in either equation to solve for y Let's choose the first one yClick here to see ALL problems on Linearequations Question 6702 Solve the following system using substitution x3y=8 2xy=2 Answer by jim_thompson5910 () ( Show Source ) You can put this solution on YOUR website!Question solve using the substitution method y = 3 x 2x 4y = 6 Answer by jim_thompson5910() (Show Source) You can put this solution on YOUR website!




Exercise 33 1 Solve The Follo See How To Solve It At Qanda



3 Systems Of Linear Equations
Substitution Method Use the Substitution method to solve the system of equations x y = 4 x y = 2 x y = 4 x y = 2 In the second equation, x= y2 Putting that in the first (y2) y= 4 subtract two from each side, then divide each side by Solve the following pair of linear equations by substitution method x y = 2m;Solve by Substitution 2xy=3 , xy=0 2x − y = −3 2 x y = 3 , x y = 0 x y = 0 Subtract y y from both sides of the equation x = −y x = y 2x−y = −3 2 x y = 3 Replace all occurrences of x x with −y y in each equation Tap for more steps Replace all occurrences of x x in 2 x − y = − 3 2 x y = 3 with − y
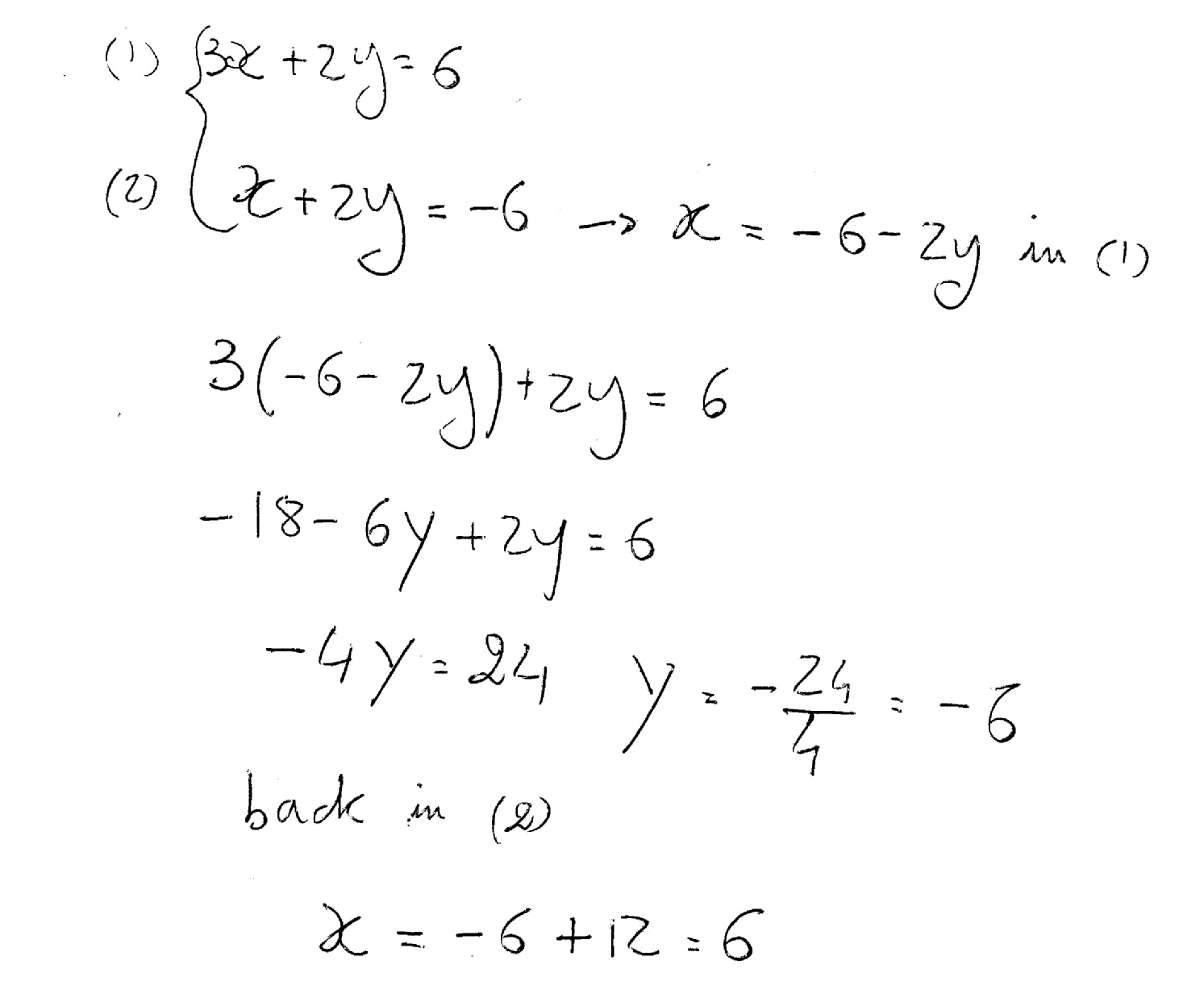



How Do You Solve By Substitution 3x 2y 6 And X 2y 6 Socratic




Systems Of Equations With Substitution 2y X 7 X Y 4 Video Khan Academy



No comments:
Post a Comment