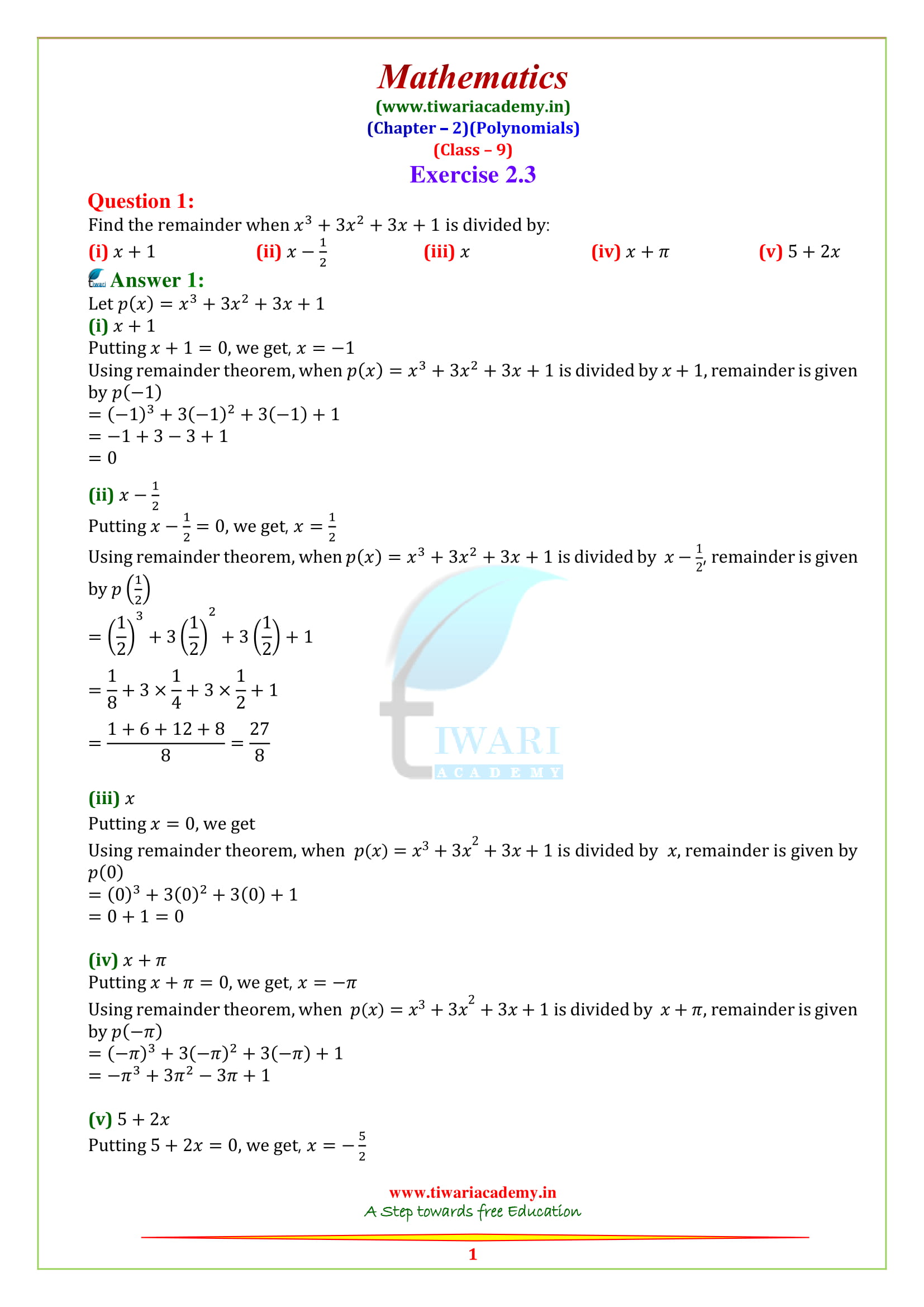
In mathematics, the logarithm is the inverse function to exponentiationThat means the logarithm of a given number x is the exponent to which another fixed number, the base b, must be raised, to produce that number xIn the simplest case, the logarithm counts the number of occurrences of the same factor in repeated multiplication; Ex 25, 7 Evaluate the following using suitable identities (i) (99)3 We write 99 = 100 – 1 (99)3 = (100 − 1)3 Using (a – b)3 = a3 – b3 – 3ab(a – b) Where a = 100 & b = 1 = (100)3 − (1)3 − 3(100) (1) (100 − 1) = − 1 − 300(99) = − 1 − = Ex 25, 7 Eva(a 3 3a 2 b 3ab 2 b 3)(ab) = a 4 4a 3 b 6a 2 b 2 4ab 3 b 4 The calculations get longer and longer as we go, but there is some kind of pattern developing That pattern is summed up by the Binomial Theorem

Qg0dbobeox2fsm
Expand (1/x+y/3)^3 class 9
Expand (1/x+y/3)^3 class 9- ML Aggarwal Class 9 Solutions for ICSE Maths Chapter 3 Expansions Chapter Test Question 1 Find the expansions of the following (i) (2x 3y 5) (2x 3y – 5)Class 9 RD Sharma Solutions Chapter 4 Algebraic Identities Ex 43 Question 1 Find the cube of each of the following binomial expressions Solution Question 2 If a b = 10 and ab = 21, find the value of a 3 b 3 Solution a b = 10, ab = 21




Rd Sharma Class 9th Solutions Chapter 1 Number Systems
Quotient of x^38x^217x6 with x3;👉 Learn all about sequences In this playlist, we will explore how to write the rule for a sequence, determine the nth term, determine the first 5 terms orTaylor series of a function is the sum of infinite series or infinite terms Taylor series is polynomial of sum of infinite degree It is used in various fields such as calculus Maclaurin Series Expansion
(),where f (n) (a) denotes the n th derivative of f evaluated at the point aBrainlycom For students By students Brainly is the place to learn The world's largest social learning network for students Explanation (x −y)3 = (x − y)(x −y)(x −y) Expand the first two brackets (x −y)(x − y) = x2 −xy −xy y2 ⇒ x2 y2 − 2xy Multiply the result by the last two brackets (x2 y2 −2xy)(x − y) = x3 − x2y xy2 − y3 −2x2y 2xy2 ⇒ x3 −y3 − 3x2y 3xy2 Always expand each term in the bracket by all the other
Write expression log(x4y7 z16) log ( x 4 y 7 z 16) as a sum or difference of logarithms with no exponents Simplify your answer completely log(x4y7 z16) = log ( x 4 y 7 z 16) = Get help Box 1 Enter your answer as an expression Example 3x^21, x/5, (ab)/c Be sure your variables match those in the questionCompute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, historyPhone support is available MondayFriday, 900AM1000PM ET You may speak with a member of our customer support team by calling End of Conversation Have a great day!




Selina Solutions For Concise Mathematics Class 9 Icse Chapter 4 Expansions Including Substitution Latest Edition Shaalaa Com




Please Expand 1 X Y 3 Whole Cube Brainly In
This calculator can be used to expand and simplify any polynomial expressionShare It On Facebook Twitter Email 1 Answer 0 votes answered by Atthar (434k points) selected by Maniraj Best answer Correct Answer CML Aggarwal Solutions for Class 9 Maths Chapter 3 – Expansions are provided here to help students prepare and excel in their exams This chapter mainly deals with problems based on expansions Experts tutors have formulated the solutions in a step by step manner for students to grasp the concepts easily From the exam point of view, solving the problems on a regular basis,




6 Div 0 2 See How To Solve It At Qanda



4 The Binomial Theorem
View more examples » Access instant learning tools Get immediate feedback and guidance with stepbystep solutions and Wolfram Problem Generator Learn Find an answer to your question if x/y y/x = 1 then find x^3 y ^3 Yar kya hai tuja Sala Daru piya hai kya Jyada Jo Bar bar ek hi message repeat Kar Rah hai yah Bhut gussa dil wah Rah hai kya karu Iska Chal tuja bakshDesmos offers bestinclass calculators, digital math activities, and curriculum to help every student love math and love learning math



What Is The Binomial Expansion Of Math 1 X 2 Math Quora



What Is The Binomial Expansion Of Math 1 X 2 Math Quora
Graph{x^33x^29x5 1459, 1726, 856, 736} FIrst determine the interval of definition, then the behavior of first and second derivatives and the behavior of the function as \displaystyle{x}NCERT Solutions for Class 9 Maths Chapter 2 has 5 exercises The topics discussed in these exercises are polynomials in one Variable, zeros of a polynomial, real numbers and their decimal expansions, representing real numbers on the number line and operations on real numbers laws of exponents for real numbersExample Expand 3 × (52) Answer It is now expanded We can also complete the calculation 3 × (52) = 3 × 5 3 × 2 = 15 6 = 21 In Algebra In Algebra putting two things next to each other usually means to multiply So 3(ab) means to multiply 3 by (ab) Here is an example of expanding, using variables a, b and c instead of numbers



Ncert Solutions For Class 9 Maths Chapter 2 Polynomials In Pdf



Find The Cube Of Each Of The Following Binomial Expressions I 1 X Y 3 Ii 3 X 2 X 2 Sarthaks Econnect Largest Online Education Community
Denotes the factorial of nIn the more compact sigma notation, this can be written as = ()!1 x 2 = (3 2i) x 1 x 2 )2ix 2 = x 1 For x 2 = 1 we obtain x(1) = (2i 1 Finally for the second eigenvalue 2, the eigenvector is x(2) = 2i i Math 331 Homework Assignment Chapter 7 Page 4 of 9 4 For the system of di erential equations x0= Ax A = 2 5 1 2 and the two vectorvalued functions x 1(t) = 5cost 2cost sint ; Ex 25, 4 Expand each of the following, using suitable identities (x 2y 4z)2 (x 2y 4z)2 Using (a b c)2 = a2 b2 c2 2ab 2bc 2ac Where a = x , b




Ncert Solutions For Class 9 Maths Chapter 2 Polynomials Ex 2 5 Cbsetuts Com




Number Of Terms In Expansion Of Following I 2x 3y 9 Ii 2



No comments:
Post a Comment